To begin testing the fundamental principles, I wanted to create something hands-on to get the feel for it. The digital world can sometimes be far removed from reality. As I began gathering various sources, I saw the principle of levels of hierarchy (or fractals) begin repeated with structure throughout nature.

The cross-section structure of corals resembles fractals at various scales
I began by sketching various self-similar structures (not necessarily inspired by nature) and while sketching, I began wondering about their physical properties. Amongst the various structures I sketched, I chose to go ahead with the hexagon honeycomb pattern. It is probably the most cliché example in a biomimicry project, but I thought it being cliché might help me. If I apply the principles correctly, I believed I could not only demonstrate the validity of the principles but also be able to highlight the difference between a top-down approach and a bottom-up approach to biomimicry. Well for that to happen, I needed the structure to work. What does it mean for a structure to work? Which context should I take? I hypothesized that the structure will be more efficient as it will use less substance and provide more rigidity to stress/strain compared to a regular honeycomb pattern. Perhaps there would be unexpected emergent properties as well? For the experiment, I needed a model to compare as well. So I decided to create a regular hexagon structure as well, so that I could feel the structure, test it and draw conclusions.
The fractal hexagon pattern at each iteration
Figuring out the length of relationships was fairly trivial. Each iteration of the fractal has its side length reduced by a factor of sqrt(3)/2. In each iteration, the hexagons are also rotated. I started construction by taking cardboard and making strips of cardboard. The strips were then creased at the right length and bent. In the end, the strips were joined together to get the hexagonal structure.
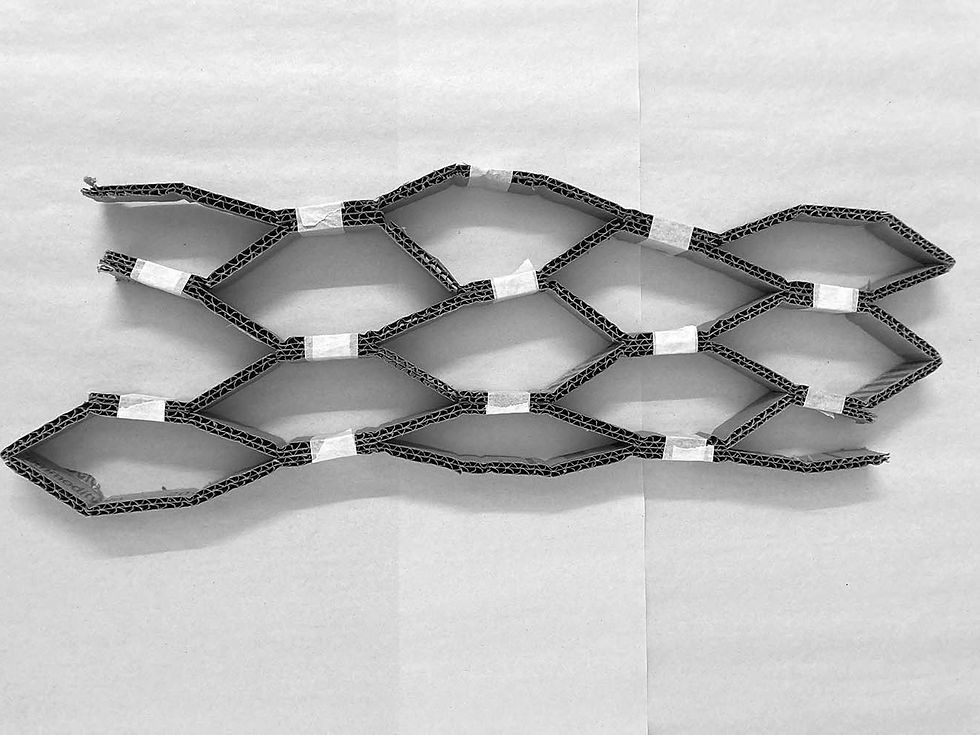
The first cardboard model
The structure was quite flimsy. The cardboard was heavy and so it was unable to support its weight. It showed a tendency to be easily squished from one direction. I then started adding the next iteration of the fractal hoping these properties would improve.
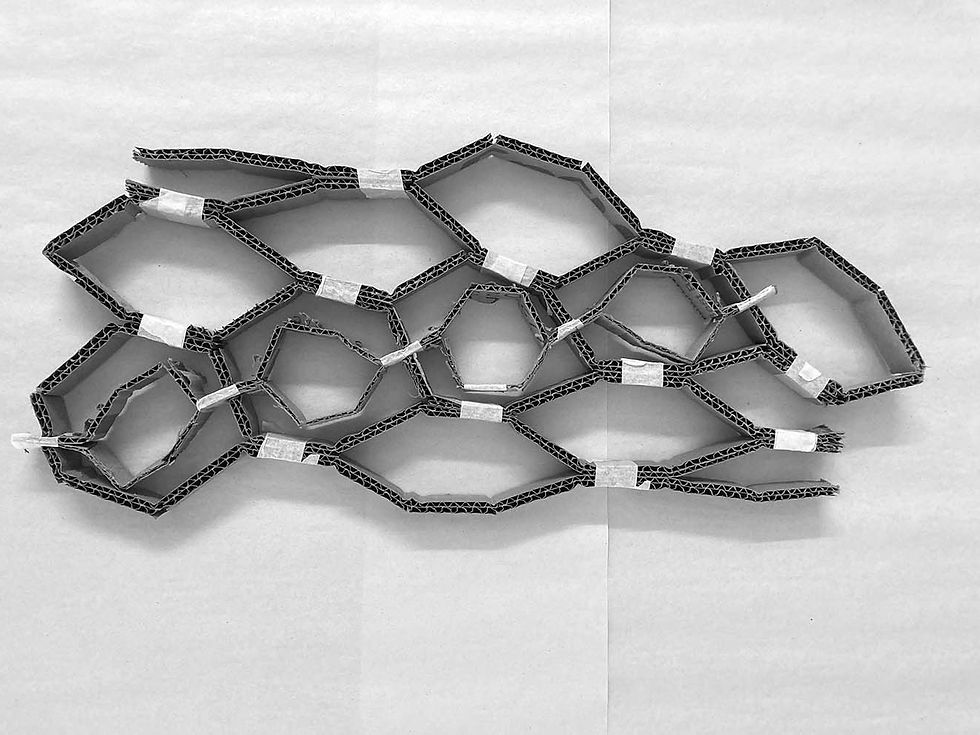
Addition of a single layer of the first iteration
With just the first layer, I saw remarkable improvements in its properties. It showed less tendency to resist stress and strain where the first iteration was added. The cardboard was getting tedious to work with. I also had to account for cardboard thickness at each stage which became a lot of redundant work for just testing out the structure. Moreover, it didn't give a nice finish. So I decided to prototype using paper.
I constructed 3 structures to compare the properties. All three use the same amount of material (i.e same mass of paper). They just differ in their structure. One is the regular hexagon grid with a side length of 3cm (so that I get the same size to compare with). The fractal structure has the first layer of a hexagon with a side length of 6cm (each iteration has the side length reduced by a factor of sqrt(3)/2). Lastly, I constructed a triangular grid as it seemed to be more rigid and is also in fact a self-similar fractal structure on its own.
Regular Hexagon (Left), Triangular Grid (Center), Fractal Hexagon (Right)
TODO: Put pictures of structures in action
As I played around with the models I reached some conclusions. The regular hexagon grid is quite flexible and can bend outside of its plane. However, it becomes difficult for it to hold up its weight. The paper strips would bend in some parts. It can distribute a force applied over a large area relatively well, but the moment a force is applied in a small area, it gets squished very easily. So it doesn't perform well under pressure. This showed a perfect analogy to the stress and strain forces that would happen if it were a steel structure. The only difference is that where paper can bend (by being flexible), the steel structure would crack and break when it would approach its limit (as defined by Young's Modulus). Thus the paper models serve as good tools to extrapolate the structure to other materials and even increase in size. (Increasing in size results in a cubic increase of mass and volume and a square increase of tensile strength).
The triangular grid is quite rigid and strong. However, for the same amount of paper required to build the regular hexagon, the triangular grid gives exactly half the size. So it can be concluded that the increase in density of the substance is what provided the increase in rigidity (though in reality some of it may be attributed to the fractal structure as well). So yes, the triangular grid is better at achieving more rigidity but at the cost of using more material for the same area and a loss in flexibility (the structure is quite stiff to bend out of the plane). Lastly, the hexagonal fractal structure gives the same amount of area while using slightly less amount of paper compared to the regular hexagon grid. It is much more stiffer and rigid (though not as much as the triangular grid). It can support its weight very well. It can distribute the force applied just like the regular hexagon grid and also can resist a force applied to a small area (pressure). Moreover, it didn't lose its ability to be flexible and bend out of the plane. Thus, it showed that the structure was a win-win in all cases - better at providing rigidity, minimum use of material, and being flexible.
Learnings
So what were the learnings and new insights? It seems that the fractal nature of the structure is like leverage. Just like how leverage can maximize (and multiply) work done from the effort put in, the fractal structure can enhance properties (effectively multiply exponentially at each iteration) for the same amount of effort (in this case substance/material) that we put in.
But the triangular grid is also a fractal. So what makes the hexagonal fractal much more efficient than the triangular grid? Is there some underlying fact about the nature of fractals that directly affects the physical properties? I suspect that the amount of leverage depends on the fractal dimension of the fractal. The more the fractal dimensions, the greater the amount of leverage. Challenges
The prototypes that I had constructed used paper and tape to make the connections. However, in practical applications, well-defined joinery would be required. The challenge is not so much in the structure but the joinery. For example, on each iteration of the fractal, components will need to be joined to each other in a different unique way. The joinery needs to be strong and lightweight such that it doesn't counteract the leverage provided by the fractal structure. We can't keep on increasing the fractal iterations because of the limitations of the joinery. How could this challenge be overcome? We would need to look at fractals differently and see how nature deals with building structures and fractals.
There are some distinct characteristics in nature's structures that stand out:
Only the local effects are considered. The entire form is emergent. Local effects and rules are followed throughout the process.
Rarely do we see perfect copies. Variation and randomness are part of the process and still, it can surpass manmade objects.
Nature rarely uses subtractive manufacturing methods. Most are based on the principle of additive manufacturing (especially ones that involve living organisms).
This gives further direction to the different types of experiments that could be performed. Also, the similarity of these insights and processes used in the indigenous manufacturing of objects is quite close. For instance, structural domes constructed by indigenous methods have precision in the process but individual elements are randomly and chaotically arranged. Yet, the emergent forms are ordered and structurally stable. Further, the entire form need not be imagined and calculated before starting to construct. Most of the processes also involve rearrangement, deposition, or growth are additive in nature.
Some topics of inquiry:
Can we have randomness and probability in the precise processes of today's manufacturing methods? How else can fractal structures be utilized, apart from load-bearing?
Flexible Materials
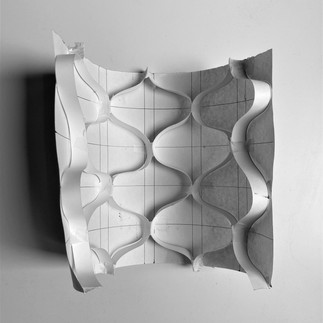
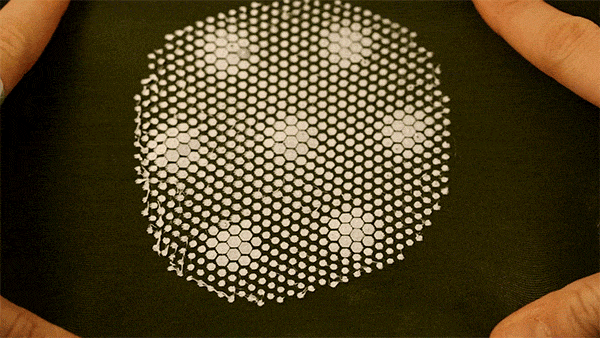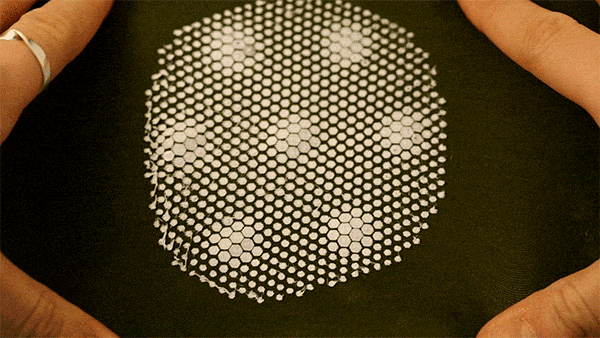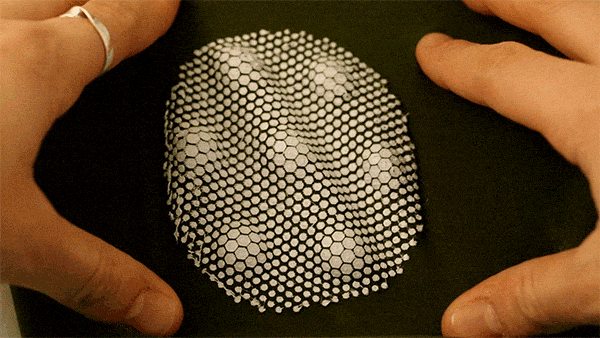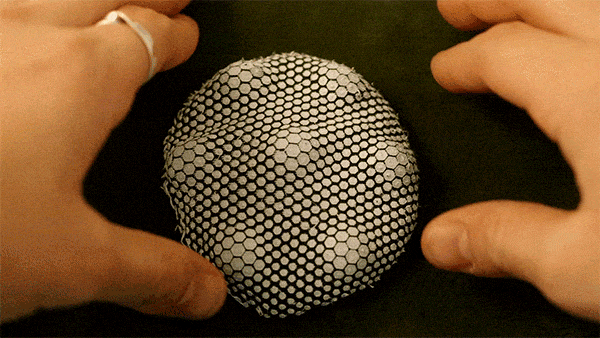
Another direction that would allow building the complex fractal is combining the rigidity of solid structures with flexible materials. For instance, 3d printing on fabric allows building complex forms that would self-assemble. Similarly, could be self-assemble fractals instead of constructing them? For some initial experiments, I laid down paper strips on paper. This would mimic depositing material on a flexible membrane. I was able to alter the curvature, shape, and movement based on the distance between elements. The solid materials provide rigidity while the flexible materials provide flexibility. By using these in combination we can leverage both the properties simultaneously.
As can be seen in the last image (from Nervous System studio), stretching of fabric would allow even more control over the self-assembly of form.



























Comments